Sinh (röd), cosh (grön) och tanh (blå). Koppling mellan hyperbler och de hyperboliska funktionerna. Varje punkt på högra delen av hyperbeln har koordinaten (cosh a, sinh a) där a är dubbla rödmarkerade arean i figuren. Inom matematiken är de hyperboliska funktionerna nära besläktade med de trigonometriska funktionerna , vilket antyds av deras benämningar:
sinus hyperbolicus (sinh)
cosinus hyperbolicus (cosh)
tangens hyperbolicus (tanh)
secans hyperbolicus (sech)
cosecans hyperbolicus (csch)
cotangens hyperbolicus (coth) sech och csch används sällan.
De hyperboliska funktionernas definitioner är
sinh
x
=
e
x
−
e
−
x
2
{\displaystyle \sinh x={\frac {e^{x}-e^{-x}}{2}}}
cosh
x
=
e
x
+
e
−
x
2
{\displaystyle \cosh x={\frac {e^{x}+e^{-x}}{2}}}
tanh
x
=
sinh
x
cosh
x
=
e
x
−
e
−
x
e
x
+
e
−
x
{\displaystyle \tanh x={\frac {\sinh x}{\cosh x}}={\frac {e^{x}-e^{-x}}{e^{x}+e^{-x}}}}
sech
(
x
)
=
1
cosh
x
=
2
e
x
+
e
−
x
{\displaystyle \operatorname {sech} (x)={\frac {1}{\cosh x}}={\frac {2}{e^{x}+e^{-x}}}}
csch
(
x
)
=
1
sinh
x
=
2
e
x
−
e
−
x
{\displaystyle \operatorname {csch} (x)={\frac {1}{\sinh x}}={\frac {2}{e^{x}-e^{-x}}}}
coth
x
=
1
tanh
x
=
e
x
+
e
−
x
e
x
−
e
−
x
{\displaystyle \coth x={\frac {1}{\tanh x}}={\frac {e^{x}+e^{-x}}{e^{x}-e^{-x}}}}
Vid jämförelse med Eulers formler , framgår att enligt definitionerna av cosh och cos är skillnaden att vinkeln är multiplicerad med komplexa enheten i ; motsvarande gäller för sin och sinh:
cosh
(
x
)
=
cos
(
i
x
)
,
cosh
(
i
x
)
=
cos
(
x
)
{\displaystyle \cosh(x)=\cos(ix),\qquad \cosh(ix)=\cos(x)}
sinh
(
x
)
=
−
i
sin
(
i
x
)
,
sinh
(
i
x
)
=
i
sin
(
x
)
{\displaystyle \sinh(x)=-i\sin(ix),\qquad \sinh(ix)=i\sin(x)}
och därmed kan de trigonometriska funktionerna – ur ett analytiskt perspektiv – betraktas som utvidgningar av de hyperboliska funktionerna till det komplexa talplanet . Ur ett geometriskt perspektiv är dock de trigonometriska funktionerna mer grundläggande och man kan då – ur denna synvinkel – betrakta de hyperboliska funktionerna som utvidgningar till det komplexa talplanet av trigonometriska funktioner.
Utveckling av sinh och cosh i en taylorserie kan göras med hjälp av serieutvecklingar av exponentialfunktionen :
sinh
(
x
)
=
∑
k
=
0
∞
x
2
k
+
1
(
2
k
+
1
)
!
cosh
(
x
)
=
∑
k
=
0
∞
x
2
k
(
2
k
)
!
{\displaystyle \sinh(x)=\sum _{k=0}^{\infty }{\frac {x^{2k+1}}{(2k+1)!}}\qquad \cosh(x)=\sum _{k=0}^{\infty }{\frac {x^{2k}}{(2k)!}}}
Motsvarigheten till trigonometriska ettan , kallad hyperboliska ettan:
cosh
2
x
−
sinh
2
x
=
1
{\displaystyle \cosh ^{2}x-\sinh ^{2}x=1}
sinh är udda , cosh är jämn :
cosh
(
−
x
)
=
cosh
x
{\displaystyle \cosh(-x)=\cosh x}
sinh
(
−
x
)
=
−
sinh
x
{\displaystyle \sinh(-x)=-\sinh x}
Summor:
sinh
(
x
+
y
)
=
sinh
(
x
)
⋅
cosh
(
y
)
+
cosh
(
x
)
⋅
sinh
(
y
)
{\displaystyle \sinh {(x+y)}=\sinh {(x)}\cdot \cosh {(y)}+\cosh {(x)}\cdot \sinh {(y)}}
sinh
(
x
−
y
)
=
sinh
(
x
)
⋅
cosh
(
y
)
−
cosh
(
x
)
⋅
sinh
(
y
)
{\displaystyle \sinh {(x-y)}=\sinh {(x)}\cdot \cosh {(y)}-\cosh {(x)}\cdot \sinh {(y)}}
cosh
(
x
+
y
)
=
cosh
(
x
)
⋅
cosh
(
y
)
+
sinh
(
x
)
⋅
sinh
(
y
)
{\displaystyle \cosh {(x+y)}=\cosh {(x)}\cdot \cosh {(y)}+\sinh {(x)}\cdot \sinh {(y)}}
cosh
(
x
−
y
)
=
cosh
(
x
)
⋅
cosh
(
y
)
−
sinh
(
x
)
⋅
sinh
(
y
)
{\displaystyle \cosh {(x-y)}=\cosh {(x)}\cdot \cosh {(y)}-\sinh {(x)}\cdot \sinh {(y)}}
De hyperboliska funktionernas inverser benämns area hyperbolicus eller arcus hyperbolicus . Dock kan varje sådan invers-funktion skrivas med hjälp av logaritmer :
arcsinh
x
=
ln
(
x
+
x
2
+
1
)
{\displaystyle \operatorname {arcsinh} x=\ln(x+{\sqrt {x^{2}+1}})}
arccosh
x
=
ln
(
x
+
x
2
−
1
)
{\displaystyle \operatorname {arccosh} x=\ln(x+{\sqrt {x^{2}-1}})}
arctanh
x
=
1
2
⋅
ln
(
1
+
x
1
−
x
)
{\displaystyle \operatorname {arctanh} x={\frac {1}{2}}\cdot \ln \left({\frac {1+x}{1-x}}\right)}
Speciellt gäller att arcsinh är entydigt definierad för hela ℝ till skillnad från inverserna av de trigonometriska funktionerna där man undviker flertydighet genom att införa begreppet principalvärde .
d
d
x
sinh
(
x
)
=
cosh
(
x
)
{\displaystyle {\frac {d}{dx}}\sinh(x)=\cosh(x)\,}
d
d
x
cosh
(
x
)
=
sinh
(
x
)
{\displaystyle {\frac {d}{dx}}\cosh(x)=\sinh(x)\,}
d
d
x
tanh
(
x
)
=
1
−
tanh
2
(
x
)
{\displaystyle {\frac {d}{dx}}\tanh(x)=1-\tanh ^{2}(x)\,}
d
d
x
coth
(
x
)
=
1
−
coth
2
(
x
)
{\displaystyle {\frac {d}{dx}}\coth(x)=1-\coth ^{2}(x)\,}
d
d
x
csch(x)
=
−
coth
(
x
)
csch(x)
{\displaystyle {\frac {d}{dx}}\ {\hbox{csch(x)}}=-\coth(x)\ {\hbox{csch(x)}}\,}
d
d
x
sech(x)
=
−
tanh
(
x
)
sech(x)
{\displaystyle {\frac {d}{dx}}\ {\hbox{sech(x)}}=-\tanh(x)\ {\hbox{sech(x)}}\,}
d
d
x
arcsinh
(
x
)
=
1
x
2
+
1
{\displaystyle {\frac {d}{dx}}\operatorname {arcsinh} (x)={\frac {1}{\sqrt {x^{2}+1}}}}
d
d
x
arccosh
(
x
)
=
1
x
2
−
1
{\displaystyle {\frac {d}{dx}}\operatorname {arccosh} (x)={\frac {1}{\sqrt {x^{2}-1}}}}
d
d
x
arctanh
(
x
)
=
1
1
−
x
2
{\displaystyle {\frac {d}{dx}}\operatorname {arctanh} (x)={\frac {1}{1-x^{2}}}}
d
d
x
arccsch
(
x
)
=
−
1
|
x
|
1
+
x
2
{\displaystyle {\frac {d}{dx}}\operatorname {arccsch} (x)=-{\frac {1}{\left|x\right|{\sqrt {1+x^{2}}}}}}
d
d
x
arcsech
(
x
)
=
−
1
x
1
−
x
2
{\displaystyle {\frac {d}{dx}}\operatorname {arcsech} (x)=-{\frac {1}{x{\sqrt {1-x^{2}}}}}}
d
d
x
arccoth
(
x
)
=
1
1
−
x
2
{\displaystyle {\frac {d}{dx}}\operatorname {arccoth} (x)={\frac {1}{1-x^{2}}}}
Hyperbolisk funktion .GonioLab : Visualisering av enhetscirkeln, trigonometriska och hyperboliska funktioner (Java Web Start)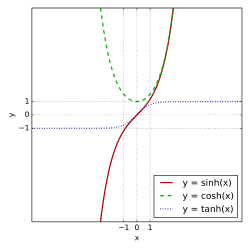

 Wikimedia Commons har media som rör Hyperbolisk funktion.
Wikimedia Commons har media som rör Hyperbolisk funktion.






























